Dr. Robin Selinger
"Theoretical/Computational Studies of Soft Matter"
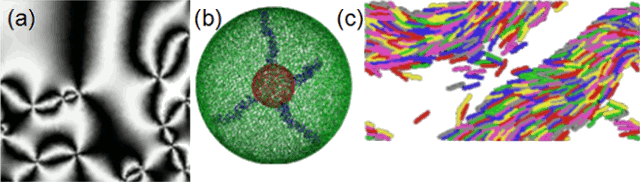
Figure 1: Simulation studies of: (a) defect texture evolution in a SC thin film; (b) LC shell-shaped droplet microstructure; and (c) a nematic LC showing giant density fluctuations. All simulations and visualizations were created by undergraduate students working with Prof. Selinger.
SelingerŌĆÖs group uses modeling/simulation to study fundamental mechanisms governing pattern formation and shape evolution in soft matter1-3 ŌłÆ such as LCs, Langmuir monolayers, colloids, and nematic elastomers. Techniques include molecular dynamics, Monte Carlo, and finite element simulation. Multiscale approaches allow simultaneous modeling of a sampleŌĆÖs macroscopic deformation together with evolution of internal microstructure.
Example projects include: (1) Study of defect pattern formation in LCs in 2D, 3D, and curved geometries ŌĆō Topological defect textures in LCs form complex patterns due to interactions with each other, with surface anchoring forces and the effects of curved geometries, and with applied electric fields. Shown in Fig. 1(a) is a frame from a dynamic stimulation of texture evolution in a SC thin film, to observe the dynamics of defect pair annihilation and the scaling exponent of the resulting coarsening behavior. Fig. 1(b) shows a Monte Carlo simulation of defect textures in a nematic "shell" confined between two spherical surfaces to compare with experimental studies.4 (2) Simulation of active nematic LCs ŌĆō unlike molecules whose thermal motion is entirely passive, self-propelled particles such as bacteria or insects form dynamic patterns which display giant density fluctuations shown in Fig. 1(c). (3) Molecular crowding ŌĆō diffusion in a solvent crowded with multiple constituents, as in a cell membrane, does not always obey FickŌĆÖs law. Using molecular dynamics simulation of diffusion in two dimensions, we explore the fundamental mechanism driving non-Fickian behavior and compare simulation results with theoretical predictions. REU students undertake projects straightforward enough to allow them to write their own simulation code with coaching by the PI and grad students, and perform their own data analysis and visualization.
- R.L.B. Selinger, A. Konya, A. Travesset, and J.V. Selinger, Monte Carlo Studies of the XY Model on Two-Dimensional Curved Surfaces, J. Phys. Chem. B 115, 13989-93 (2011).
- B.L. Mbanga, F. Ye, J.V. Selinger, and R.L.B. Selinger, Modeling elastic instabilities in nematic elastomers, Phys. Rev. E 82, 051701 (2010) [4 pages].
- R.L.B. Selinger, J.V. Selinger, A. Molanoski, and J.M. Schnur, Shape selection in chiral self-assembly, Phys. Rev. Lett. 93, 158103 (2004) [4 pages].
- T. Lopez-Leon, A. Fernandez-Nieves, M. Nobili, and C. Blanc, Nematic-smectic transition in spherical shells, Phys. Rev. Lett. 106, 247802 (2011) [4 pages].
